An optimal linear system approximation of nonlinear fractional-order memristor-capacitor charging circuit

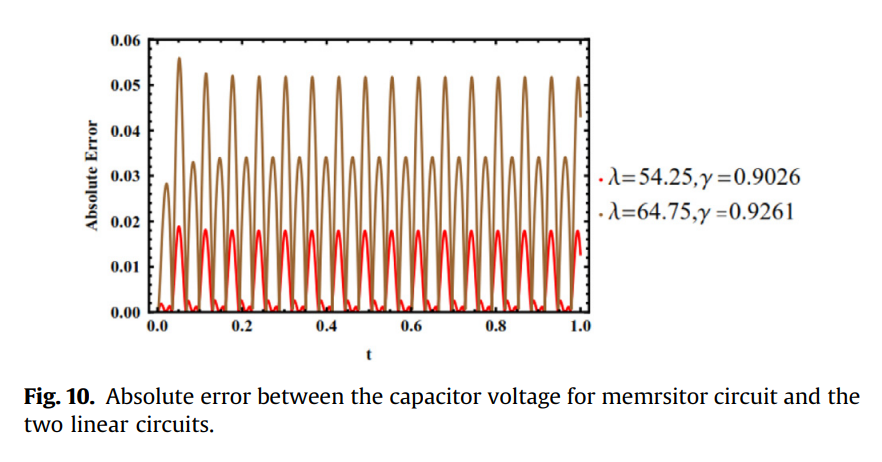
The analysis of nonlinear fractional-order circuits is a challenging problem. This is due to the lack of nonlinear circuit theorems and designs particularly in the presence of memristive elements. The response of a series connection of a simple resistor with fractional order capacitor and its analytical formulation in both charging and discharging phases is considered. The numerical simulation of fractional order HP memristor in series with a fractional order capacitor is also discussed. It is a demonstration of a simple nonlinear fractional-order memristive circuit in both charging and discharging cases. Furthermore, this paper introduces an approach to approximate nonlinear fractional-order memrisitve circuits by linear circuits using a minimax optimization technique. Hence, the new circuit can be analyzed using the conventional linear circuit theorems. The charging and discharging of a series fractional-order memristor with a fractional-order capacitor are discussed numerically. The effect of fractional-order parameters and memristor polarity are also investigated. Using a suitable optimization technique, an accurate approximation by a circuit that include a resistor and a fractional-capacitor is obtained for both charging and discharging cases. A great matching was observed between the frequency responses of the fractional-order nonlinear low pass filter based on fractional-order memristor and fractional-order capacitor and that of the optimized linear fractional order case. Similar matching is observed for the nonlinear and optimized cases when a periodic triangular waveform is applied using Fourier series expansion. © 2016 Elsevier Ltd. All rights reserved.