Breadcrumb
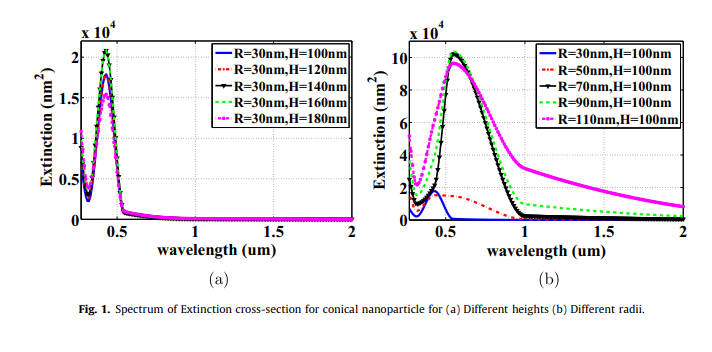
J-V characteristics of plasmonic photovoltaics with embedded conical and cylindrical metallic nanoparticles
Plasmonic photovoltaics (PVs) are promising structures that improve thin-film photovoltaics performance, where optical absorption is improved via embedding metallic nanoparticles in the PV's active layer to trap the incident optical wave into the photovoltaic cell. The presented work investigates the design of PV with both structures of conical and cylindrical metallic nanoparticles through studying their extinction cross-sections and electric field distributions. Also, the impact of these nanoparticles in silicon PVs on the optical absorption enhancement is investigated. The figure of merit
Further experimental evidence of the fractional-order energy equation in supercapacitors
Due to the dispersive porous nature of its material, carbon–carbon supercapacitors have a current–voltage relationship which is modeled by a fractional-order differential equation of the form i(t)=Cα[Formula presented] where α≤1 is a dispersion coefficient and Cα is a pseudo-capacitance not measurable in Farads. Hence, the energy stored in a capacitor, known to equal CV2/2 where C is the capacitance in Farad and V is the voltage applied, does not apply to a supercapacitor. In a recent work (Allagui et al., 2016), a fractional-order energy equation that enables the quantification of the energy
Fuzzy firefly clustering for tumour and cancer analysis
Swarm intelligence represents a meta-heuristic approach to solve a wide variety of problems. Searching for similar patterns of genes is becoming very essential to predict the expression of genes under various conditions. Firefly clustering inspired by the behaviour of fireflies helps in grouping genes that behave alike. Contrasting hard clustering methodology, fuzzy clustering assigns membership values for every gene and predicts the possibility of belonging to every cluster. To distinguish highly expressed and suppressed genes, the research in this paper proposes an efficient fuzzy-firefly
Fractional-order mathematical model for Chronic Myeloid Leukaemia
This paper is dedicated to develop a fractional order model of the rate of change of cancerous blood cells in Chronic Myeloid Leukaemia using fractional-order differential equations as well as tackling the factors that affect this rate and compare between them. The simulated cases (using MATLAB) prove that the proposed model is doable in terms of the variables positions in the equations and its effect on the overall population. Also, the effect of the Pactional order is investigated through three parameters sets and it has shown strong influence on the dynamic response. © 2017 IEEE.
Comparative study of fractional filters for Alzheimer disease detection on MRI images
This paper presents a comparative study of four fractional order filters used for edge detection. The noise performance of these filters is analyzed upon the addition of random Gaussian noise, as well as the addition of salt and pepper noise. The peak signal to noise ratio (PSNR) of the detected images is numerically compared. The mean square error (MSE) of the detected images as well as the execution time are also adopted as evaluation methods for comparison. The visual comparison of the filters capability in medical image edge detection is presented, that can help in the diagnosis of
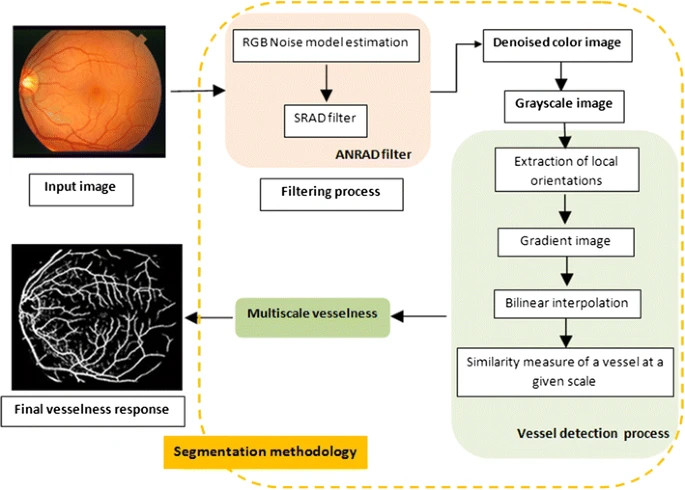
Noise-estimation-based anisotropic diffusion approach for retinal blood vessel segmentation
Recently, numerous research works in retinal-structure analysis have been performed to analyze retinal images for diagnosing and preventing ocular diseases such as diabetic retinopathy, which is the first most common causes of vision loss in the world. In this paper, an algorithm for vessel detection in fundus images is employed. First, a denoising process using the noise-estimation-based anisotropic diffusion technique is applied to restore connected vessel lines in a retinal image and eliminate noisy lines. Next, a multi-scale line-tracking algorithm is implemented to detect all the blood

A current-mode system to self-measure temperature on implantable optoelectronics
Background: One of the major concerns in implantable optoelectronics is the heat generated by emitters such as light emitting diodes (LEDs). Such devices typically produce more heat than light, whereas medical regulations state that the surface temperature change of medical implants must stay below + 2 °C. The LED's reverse current can be employed as a temperature-sensitive parameter to measure the temperature change at the implant's surface, and thus, monitor temperature rises. The main challenge in this approach is to bias the LED with a robust voltage since the reverse current is strongly
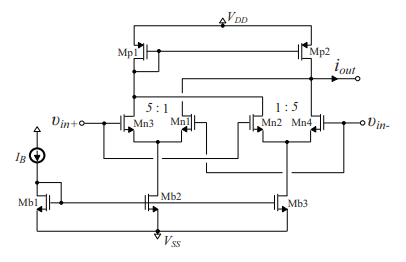
Design of fractional-order differentiator-lowpass filters for extracting the R peaks in ECG signals
An implementation of a fractional-order differentiator-lowpass filter is presented in this work, which is constructed from Operational Transconductance Amplifiers as active cells. This offers the benefits of electronic tuning and, also, of monolithic implementation. The presented scheme has been employed for the extraction of the R peaks in electrocardiogram signals due to its efficiency for performing this task even in a noisy environment. The provided post-layout simulation results confirm the correct operation of this solution as well as its reasonable sensitivity characteristics. © 2019
Simple implementations of fractional-order driving-point impedances: Application to biological tissue models
A novel procedure for the circuit implementation of the driving-point impedance of frequency-domain material models, constructed from fractional-order elements of arbitrary type and order, is introduced in this work. Following this newly introduced concept, instead of emulating separately each fractional-order element in the model under consideration, the direct emulation of the complete model can be achieved through the approximation of the total impedance function. The magnitude and phase frequency responses of the impedance function are first extracted and approximated through curve-fitting

Mathematical analysis of gene regulation activator model
This paper presents a complete analysis of the mathematical model of the gene regulation process. The model describes the induced gene expression under the effect of activators. The model differential equations are solved analytically, and the exact solution of the gene model is introduced. Moreover, a study of the model dynamics, including the fixed points and stability conditions are presented. The parameters effects on the phase plane portraits and the transient responses of the mRNA as well as the protein concentrations are intensively detailed. This work serves as a brick stone towards a
Pagination
- Page 1
- Next page ››
